The Metropolitan Museum of Art in New York City—known worldwide and hereinafter as "the Met"—is the largest art museum in the United States, and one of the ten largest in the world. Founded in 1870, it holds two million works of art, from antiquity to today, from all around the world.
We propose here a guided tour of the Met's hidden math. The Met's collections include works of art with surprising mathematical content. These works span the globe, human history, and the Met's curatorial departments. They include numerals, shapes, perspective, astronomy, time, and games. (On a second tour, still in the planning stages, we hope to show you patterns, symmetries, and various mathematical activities.)
Our tour was inspired by the late Dr. David Mininberg, whose fascinating tour "Medicine at the Met" revealed the medical purposes of jars, bowls, and other artifacts from Ancient Egypt, Byzantium, Ancient Near East, and Oceania, displayed at the Met for their artistic merit. We dedicate this essay to his memory, with gratitude for his encouragement.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
Note: We have organized this tour thematically, not by curatorial department or gallery or any other scheme, to make it easy on your feet. If you take this tour by reading it (in print or online), then you're all set to go. If you take the tour in person, you'll need to plot your path. For help with that, see our notes "If You Go" and "Where to Find It" (among these pages).
Numerals
Although this is a tour of hidden math, let's start with a 3-foot high exception. In the wing for Modern and Contemporary Art, the painting "I Saw the Figure 5 in Gold" jumps off the wall at you; or, rather, rushes away from you (Fig. 1).

Figure 1. "I Saw the Figure 5 in Gold" Charles Demuth (American, 1883–1935), 1928. Alfred Stieglitz Collection, 1949. Accession number 49.59.1. http://www.metmuseum.org/.
The painting is a tribute to the artist's friend, the poet William Carlos Williams (look for "Bill," "Carlos," and the poet's initials) and to Williams' poem, "The Great Figure":
Among the rain/and lights/I saw the figure 5/in gold/
on a red/firetruck/moving/tense/unheeded/to gong clangs/siren howls/
and wheels rumbling/through the dark city.
Next we go to Drawings and Prints. Here—less obviously—we find numerals in the mysterious, intriguing engraving "Melencolia I," made by Albrecht Durer (1471–1528) in 1514 (Fig. 2). Its 500th anniversary was heartily celebrated by mathematicians in 2014.

Figure 2. Left: Albrecht Dürer, Melencolia I, 1514. Engraving. Harris Brisbane Dick Fund, 1943. Accession number 43.106.1. http://www.metmuseum.org/. Right: Detail (the magic square).
Indeed, "Melencolia" is a mathematical feast, with a sphere, a compass, and a mysterious polyhedron. And look closely below the bell: the square with the numbers 1, . . . . 16 is a magic square: the numbers in each row, each column, and each diagonal add to 34. Although magic squares can be traced back some 2500 years, Durer's is thought to be the first European artist to depict one.
Pondering "Melencolia," you enter a wonderful world of arithmetic puzzles. What is the smallest magic square (2x2? 3x3? 4x4?) How many of each (possible) size exist? Is there a largest size? Are there any magic cubes? Magic hypercubes?
The graceful numerals in this magic square—much like the ones we use today—evolved from the ancient Brahmi numerals of India. Augmented by the tantalizing and multipurpose zero, they diffused westward slowly throughout the centuries, reaching Italy by the medieval era where they soon replaced the cumbersome Roman numerals then in use.
But another set of symbols then used in the west was not replaced so quickly. Instead it was used into the Renaissance, coexisting with the "new" Hindu-Arabic numerals, much as typing and word processing coexisted until the touch screen.
These symbols were not written; they were gestures made by flexing finger joints. Rather than simply holding up one, two, three, or more fingers to count from one to ten on two hands, hand-reckoners, as adepts were called, could display all the numbers from one to a hundred with the fingers of just one hand. With two hands, they could tally up to 9999. And not just tally: they could reckon. Hand-reckoners did arithmetic and calculated future dates of holidays such as Easter. This system probably persisted as long as it did because it was the international language of traders. A Middle Eastern camel merchant could strike a bargain with a European wool merchant without exchanging a word of their mutually incomprehensible languages, or putting pen to paper (both difficult to come by). Indeed, traders in the Chicago Board of Trade used a form of hand-reckoning ("open outcry") from 1870 to February 2015.
You can see hand reckoning at the Met in the Flemish Primitive Adriaen Isenbrant's "Man Weighing Gold" (Fig. 3), in the department of European Paintings 1250–1800. This early 16th-century painting, the Met website tells us, is one of the first portraits depicting a professional activity, although historians aren't sure just which profession is depicted. The man weighing gold may have handled commodities, or he may have been a moneychanger or a banker.
The pan on his left holds a fixed weight; he piles coins on the right-hand pan until they balance. But how does he keep track of the total? Not with an abacus and not with a pen. But notice the crossed fingers of his right hand.

Figure 3. Left: "Man Weighing Gold," Adriaen Isenbrant (Netherlandish), 1515–1520. The Friedsam Collection, Bequest of Michael Friedsam, 1931. Accession Number 32.100.36. http://www.metmuseum.org/. Right: Detail.
If our interpretation is correct, he is using a variant of the hand positions recorded by his contemporary, Luca Pacioli (Fig. 4), to tally the running sum.
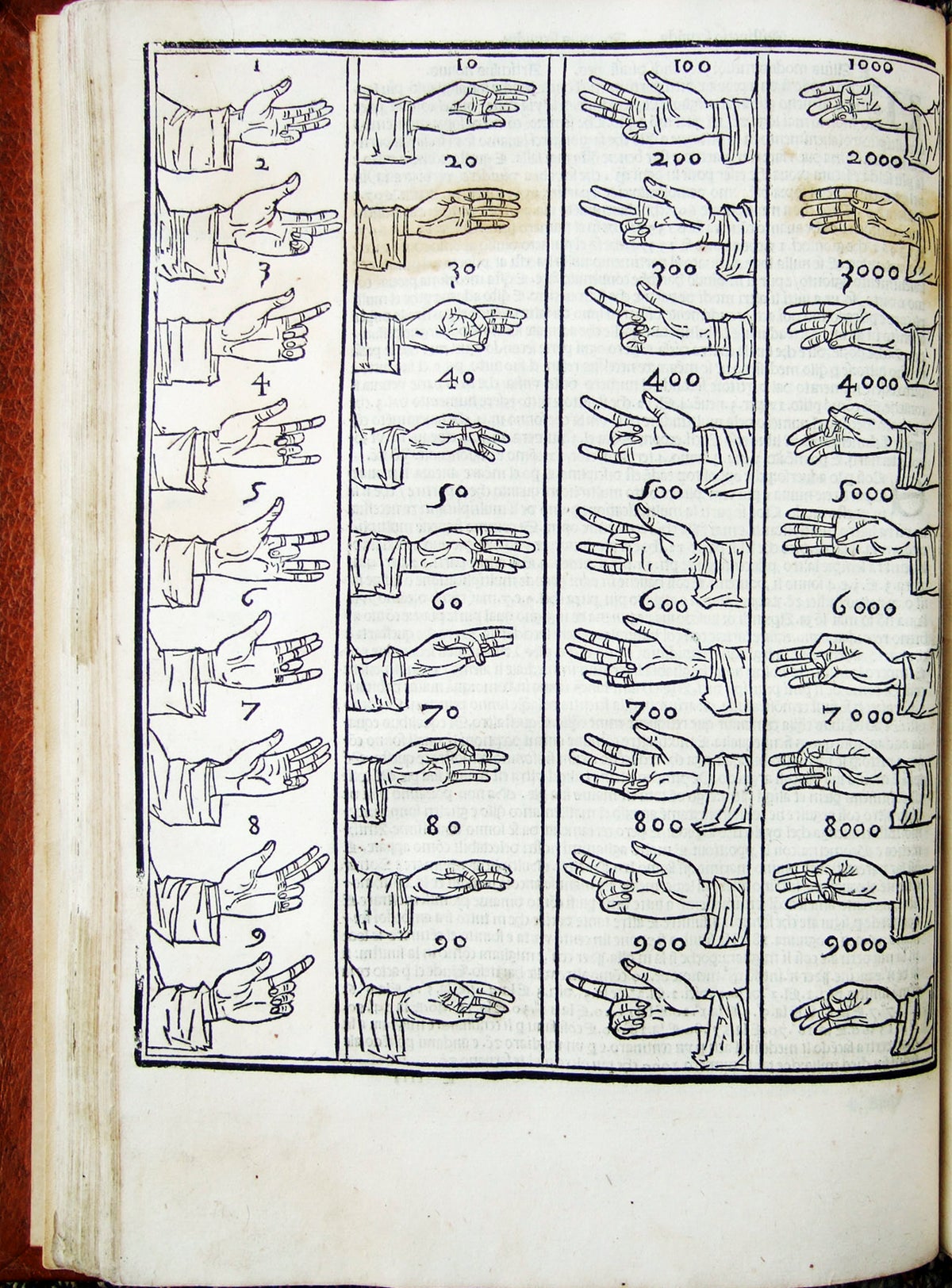
Figure 4. Finger positions for the numbers 1 to 10,000. From Luca Pacioli,
Summa de arithmetica, geometria. Proportioni et proportionalita, Venice, 1494.
In the department of European Sculpture and Decorative Arts, we find hand-reckoning again, now in an elaborate mirror frame designed by a leading Nuremburg goldsmith of the 16th century, Wenzel Jamnitzer (Fig. 5). The frame replicates the frontispiece of his 1568 book Perspectiva Corporum Regularium. Each corner represents one of the medieval university's seven liberal arts; these four, known as the quadrivium, are (clockwise from upper right) geometry, architecture, perspective drawing, and arithmetic. Look closely at "Arithmetic," upper left: the seated female figure is making the sign for the number 36 with her left hand and writing Hindu-Arabic numerals with her right.

Figure 5. Left: Relief mounted as a mirror frame, Wenzel Jamnitzer, ca. 1568. Gilded silver, ebony, mirror plate, height 11 × width 9 inches. Gift of J. Pierpont Morgan, 1917. Accession Number 17.190.620. http://www.metmuseum.org/. Right: Upper left detail (from Perspectiva Corporum Regularium). "Arithmetica" makes the hand-reckoning symbol for 36 with her left hand and writes the Hindu-Arabic numerals with her right.
Next, in the department of Asian Art, we stop before a curious terracotta tile (Fig. 6). This tile, baked in Kashmir in the 5th to 6th centuries, had been placed in an outdoor courtyard. The curious emaciated human figures in relief intrigue art historians, but the incised markings are our interest here (The markings have nothing to do with the human figures, apparently; the Met's website suggests they may indicate where in the courtyard the tile was to be placed.)
The markings on this tile are numerals, but not ones we easily recognize (except perhaps ||| for 3). Nor are these the Brahmi predecessors of today's Hindu-Arabic numerals. These numerals are written in the ancient Kharosthi script, a contemporary of the Brahmi. The cane-shaped symbol is a ten; the cross means four. Scribes and merchants in northern India and neighboring regions (and on the Silk Road) used Kharosthi numerals (Fig. 7) from about the 5th century bce to the 3rd century ce.

Figure 6. Left: Terracotta "Tile with Impressed Figure of Emaciated Ascetics and Couples Behind Balconies," 5th to 6th centuries, India (ancient kingdom of Kashmir, Harwan). Purchase, Kurt Berliner Gift, 1998. Accession Number 1998.122; http://www.metmuseum.org/. Right: The Kharosthi numerals. From K. Menninger, Number Words and Number Symbols.
We also find numerals hidden in the Egyptian Art wing, for example in an elaborate necklace and pectoral made of gold, carnelian, lapis lazuli, turquoise, garnet (pectoral), and feldspar to honor the 12th dynasty pharaoh Senwosret II (Fig. 7).

Figure 7. Left: Pectoral and Necklace of Sithathoryunet with the Name of Senwosret II, Middle Kingdom Dynasty, about 1887–1878 bce. Purchase, Rogers Fund and Henry Walters Gift, 1916. Right: The pectoral. Accession Number 16.1.3; http://www.metmuseum.org/.
The Met's translation of the hieroglyphic inscription reads, "The god of the rising sun grants life and dominion over all that the sun encircles for one million one hundred thousand years (i.e., eternity) to King Khakheperre (Senwosret II)."
And indeed the Egyptian numerals used at that time to denote a million and one hundred thousand hold center stage: the seated man is the symbol for a million, and one hundred thousand (the tadpole) dangles from his arm (Fig. 7).
Games
Still in the Egyptian wing, we find (Fig. 8) numbers represented by pips on dice from Middle Egypt, from Oxyrhynchus (el-Bahnasa), dating to the Roman Period, that is, 30 BCE–330 CE (Oxyrhynchus is best known to mathematicians for the discovery there of fragments of the most ancient version of Euclid’s Elements on papyrus, including diagrams, dating to about 100 CE, and for the vast trove of astronomical and astrological papyri recovered from the site during the past century or so, including texts, tables, ephemerides, almanacs, and horoscopes (Jones 1999)).
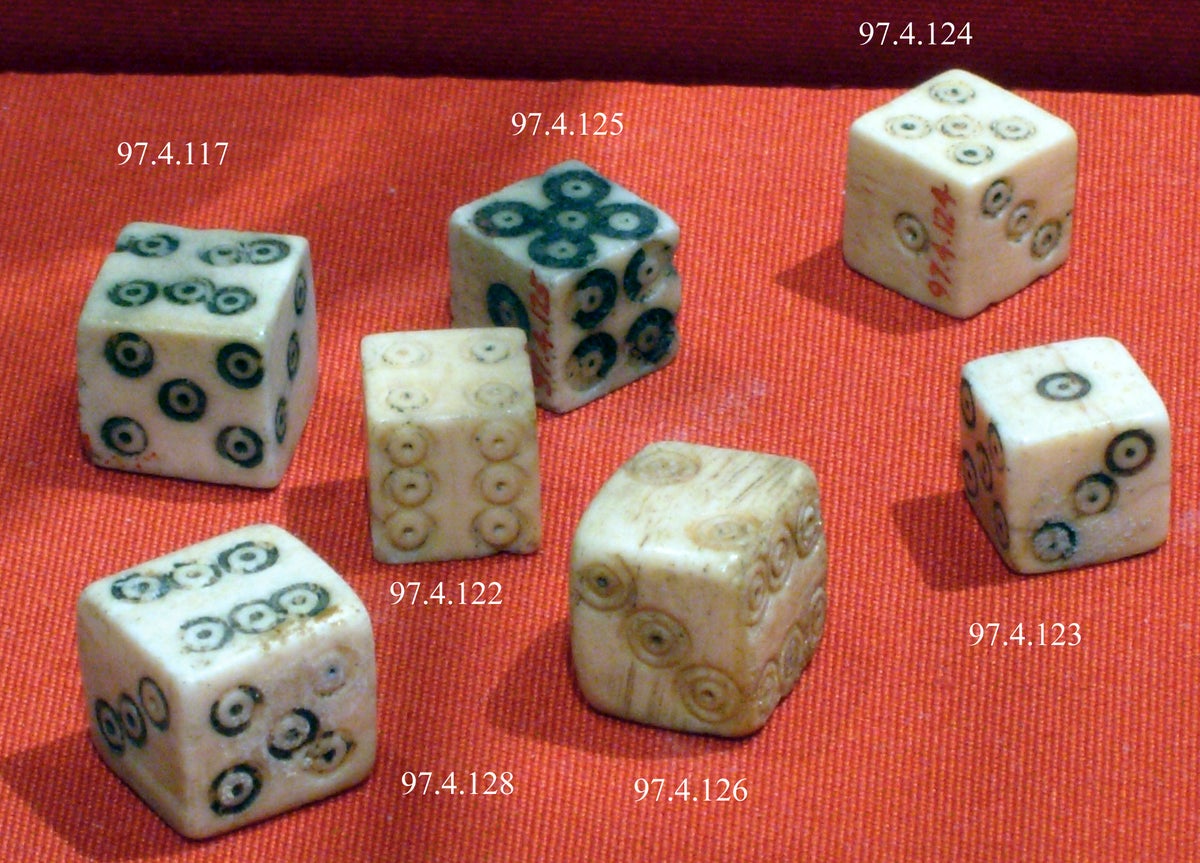
Figure 8. Dice from Oxyrhynchus, made of ivory, dimensions approximately 1 cm ( inch) on each side. Gift of the Egypt Exploration Fund, 1897. Accession Number 97.4.117. http://www.metmuseum.org/.
These dice look very much like the ones we use today. Not all ancient dice did. Other polyhedra, for example icosahedra (twenty equilateral triangular facets, grouped together in fives), were also used as dice in games of chance. In its wing for Greek and Roman Art, the Met has several examples of such Roman icosahedra (Fig. 9).

Figure 9. Icosahedra inscribed with letters of the Greek alphabet, Roman, Midimperial, 2nd to 3rd centuries. http://www.metmuseum.org/. Left: Steatite, Fletcher Fund, 1927. Accession number 27.122.5. Right: Faience, Fletcher Fund, 1937. Accession number 37.11.3.
These icosahedra were, the Met suggests, used in connection with oracles. But, because the ancient Greeks used letters of their alphabet as numerals, they may have been used as dice in games as well.
Sheep and goat knucklebones, or molded models thereof (Fig. 10), were also used as dice in certain games (Knucklebones were also used in games much like jacks). The side on which they landed when thrown determined their “value”.

Figure 10. Eight astragaloi (astragali) (Greek for knucklebones), from the Greek Hellenistic period, made of cast glass, two-part molds, averaging about 1.5 cm in height. The Gift of Mr. and Mrs. Jonathan P. Rosen, 1992. Accession Number 1992.266.3–.10. http://www.metmuseum.org/.
Games with dice were a favorite pastime in ancient Egypt. Some of them are represented in board games in the Met’s collections. This example (Fig. 11, left) of the popular board game, Game of Hounds and Jackals, is constructed of ebony and ivory. It is dated ca. 1814–1805 bce. The game was played with five pins with hounds’ and jackals’ heads. The board itself (Fig. 11, right) contains 58 holes (29 on either side of a palm tree incised down the center of the board), with the symbol of a shen ring at the center (a symbol of eternity or infinity; it could also be taken to represent the course of the Sun in the heavens).

Figure 11. Left: Game of Hounds and Jackals, Middle Kingdom, Dynasty 12, reign of Amenemhat IV, from Thebes, made of ebony and ivory, approximate dimensions (height × width × length) with pins: 14 × 10.1 × 15.6 cm. Gift of Edward S. Harkness, 1926. Accession Number 26.7.1287a–k. http://www.metmuseum.org/. Currently on display in Gallery 111. Right: Diagram of the game board (from Carter and Carnarvon).
Howard Carter and the Earl of Carnarvon excavated this board at Thebes in Upper Egypt in 1910, according to whom the game was most likely played with moves of the hounds and jackals determined by the throw of dice or knucklebones (Carter and Carnarvon 1912). “Presuming the ‘Shen’ sign…to be the goal, we find on either side twenty-nine holes, or including the goal, thirty aside. Among these holes, on either side, two are marked nefer, ‘good’; and four others are linked together by curved lines (see Fig. 14). Assuming that the holes marked ‘good’ incur a gain, it would appear that the others, connected by lines, incur a loss. Taking this for granted, and that the play terminates at the goal ‘Shen,’ the game seems then to commence at the heart of the palm—the only place where five playing pieces aside could be placed without clashing with the obstacles (i.e., holes incurring gain or loss). …Now the moves themselves could easily have been denoted by the chance cast of knuckle-bones or dice, both being known to the ancient Egyptians at an early period; and if so we have before us a simple, but exciting, game of chance” (Carter and Carnarvon 1912).
Another board game at the Met (Fig. 12), also from Thebes, dates slightly later to 1635–1450 bce, and includes gaming pieces and knucklebones presumably used in the course of the game.
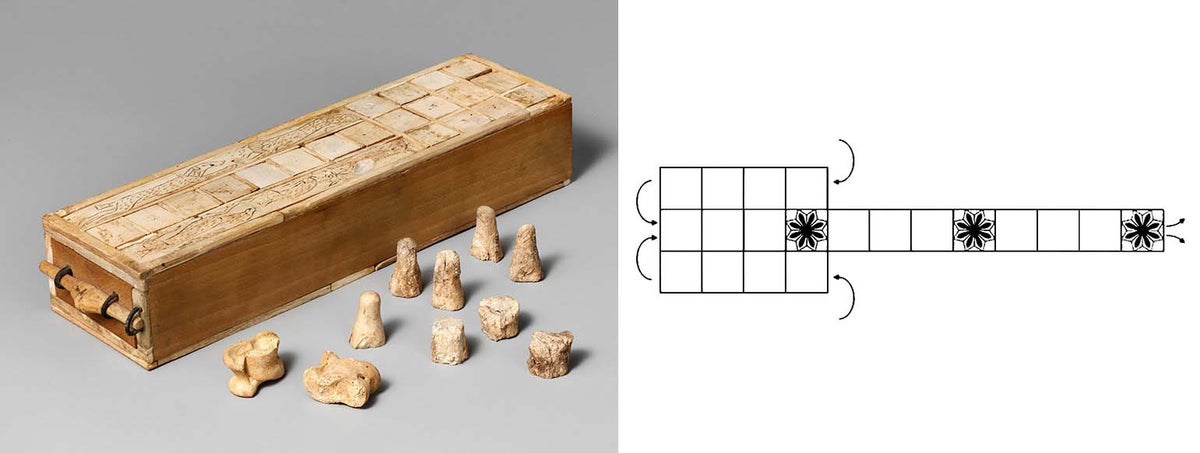
Figure 12. Left: Double-sided game box with playing pieces and a pair of knucklebones. Thebes, Egypt. ca. 1635–1458 bce. The Metropolitan Museum of Art, New York, Rogers Fund, 1916. Accession Number 16.10.475a. http://www.metmuseum.org/. Right: Diagram suggesting the direction of play for the Game of Twenty Squares (Finkel 2007).
Referred to as the “Game of Twenty Squares” or as the “Royal Game of Ur” (because of the many examples of the game excavated from locations in ancient Sumeria), the game began (Finkel 2007) with two players moving pieces toward the center aisle, as depicted in Figure 12 (right). Every fourth square is marked with a rosette or other geometric symbol. Videos on the Harvard Semitic Museum’s website, including a simplified version suitable for children, explain how players would move their game pieces depending on the throws of astragaloi or knucklebones.
Card games are represented in the Met’s collections, too. One of the best known paintings of these games is Paul Czanne’s “Les Quatres joueurs de cartes” (The Four Card Players) in the department of 19th and Early 20th Century European Paintings and Sculpture. “Les Quatres joueurs de cartes” is one of a series of five paintings that Czanne devoted to peasants playing cards. His models were local farmhands at the Jas de Bouffan, the family’s country home in Provence (Fig. 13). Notice that the legs of the central card player form the Roman numeral V. That's not incidental, says art historian Mary Louise Krumrine (Krumrine 1997): each painting in this series of five includes an instance of the number five in some form.

Figure 13. “Les Quatres Joueurs de Cartes,” Paul Cézanne, oil on canvas, painted ca. 1890–1892. Bequest of Stephen C. Clark (1960). Accession Number: 61.101.1. http://www.metmuseum.org/.
The Met also includes examples of actual decks of cards, works of art in themselves. One of these is on exhibit from time to time at the branch of the Metropolitan Museum devoted to European medieval art, The Cloisters, in Fort Tryon Park (northern Manhattan). This set of fifty-two Dutch playing cards (Fig. 14) is the only known complete set from the 15th century. Instead of the familiar diamonds, spades, clubs, and hearts, the four suits here are represented by familiar items of the hunt: dog collars, hound tethers, game nooses, and hunting horns.
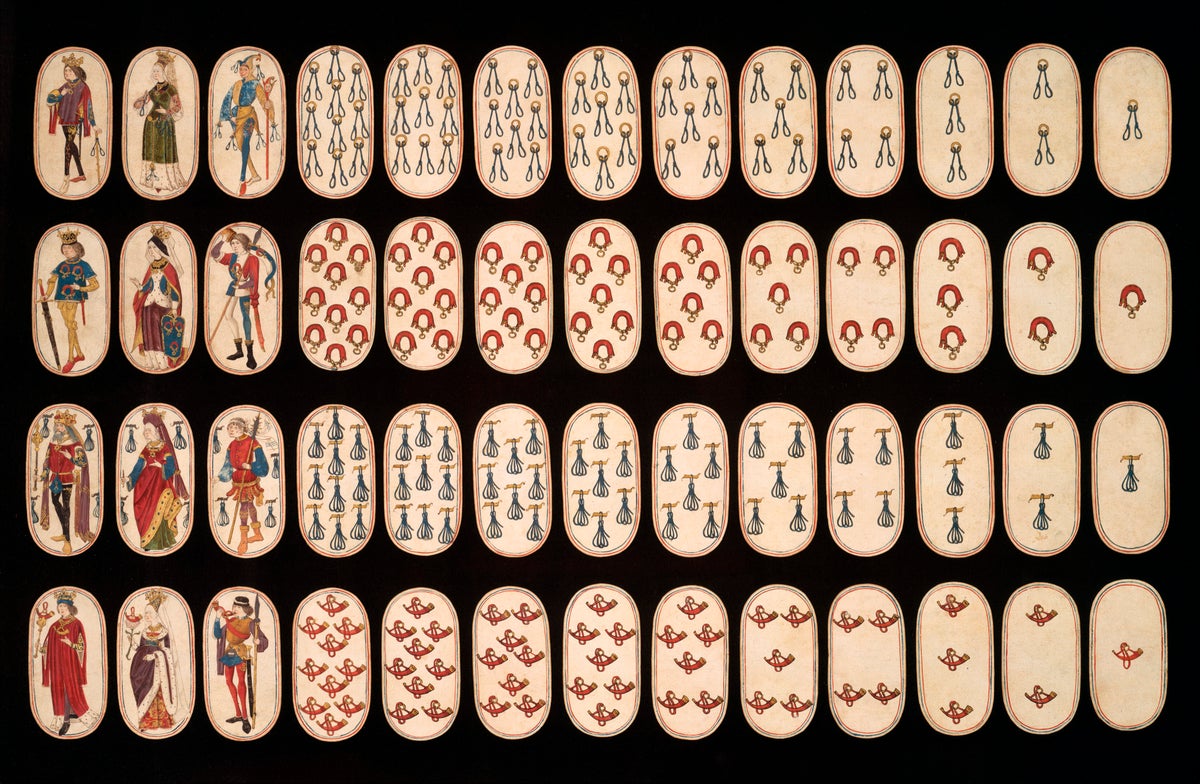
Figure 14. Playing cards, handmade, of pasteboard, pen, ink, tempera, applied gold and silver, each approximately 13.7 × 7 cm. Accession Number 1983.515.1–2. http://www.metmuseum.org/.
Dimensions
Continuing our search for hidden math, we return to the wing of Modern and Contemporary Art for Josef Albers's "Homage to the Square: With Rays." This is one in a series of works called "Homage to the Square" that Albers began in 1950. The series grew, the Met's website tells us, into "a body of more than a thousand works executed over a period of twenty-five years, including paintings, drawings, prints, and tapestries." Most depict "several squares, which appear to be overlapping or nested within one another." For Albers, the artist, the series was less about squares than colors, and how they change when we juxtapose them with others.
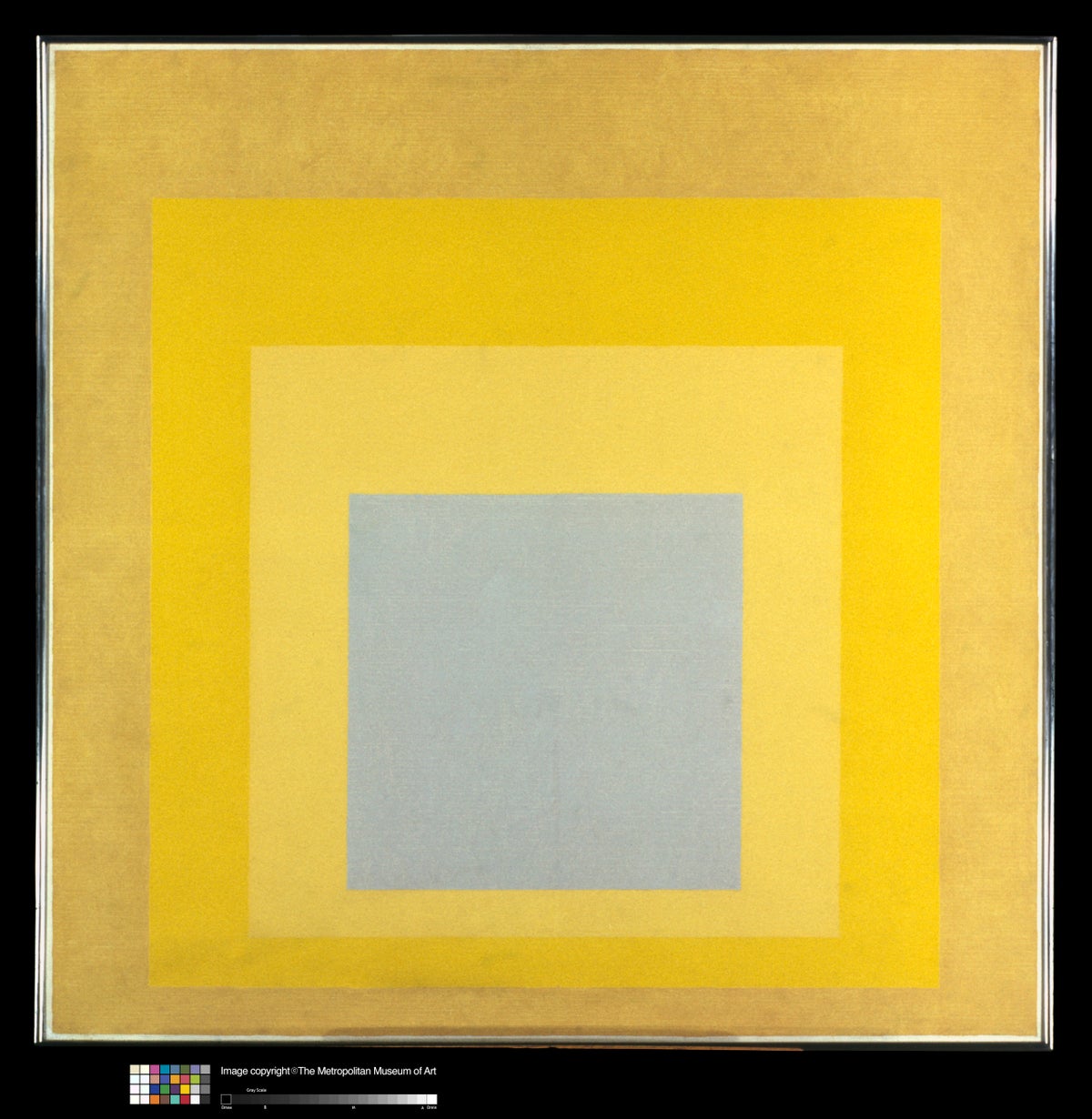
Figure 15. Homage to the Square: With Rays. Josef Albers, 1959. Oil on Masonite, 48 × 48 inches, Arthur Hoppock Hearn Fund, 1959. Accession number 59.160. ©2015 The Josef and Anni Albers Foundation/Artists Rights Society (ARS), New York.
But there's more math here than meets the eye. The remarkable color effects of the "Homage to the Square" series are discussed ad infinitum on the Internet, but Albers's "mathematically determined format" of the overlapping squares is not. The New York Times to the contrary, Albers's squares are not concentric.Can you find a mathematically precise description of the relation among the four squares in Figure 15? And what can you say about the other works in this series on the Met's website?
Moving to the Photography wing and up one dimension, consider "Sugar Cubes," a photograph by Edward Steichen (1879–1973). In this photograph (Fig. 16), we see a nearly rectangular array of ordinary sugar cubes. It's not the cubes themselves that draw and hold our attention; it's their shadows. Where did Steichen position his lamps to create this plaid effect? Test your answer experimentally, with sugar cubes from your neighborhood grocery store, and an ordinary flashlight.
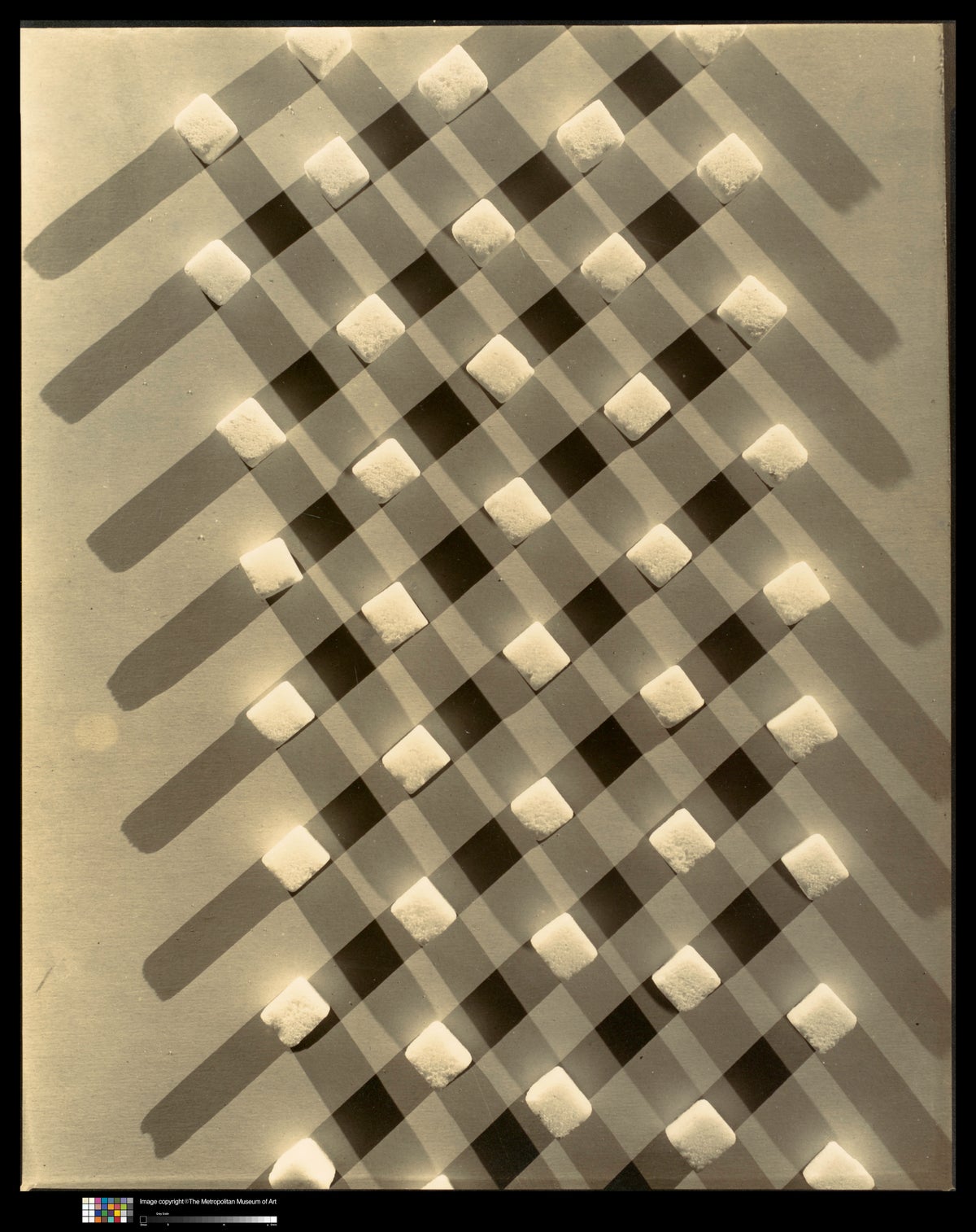
Figure 16. Sugar Cubes: Design for Stehli Silk Corporation, Edward J. Steichen, 1920s, Gelatin silver print, 9 15/16 × 8 inches. Ford Motor Company Collection, Gift of Ford Motor Company and John C. Waddell, 1987. Accession number 1987.1100.217. ©2015 The Estate of Edward Steichen/Artists Rights Society (ARS), New York.
The sugar cubes and their shadows may remind you of cubes "unfolded" to planar "nets" of squarest (Fig. 17).
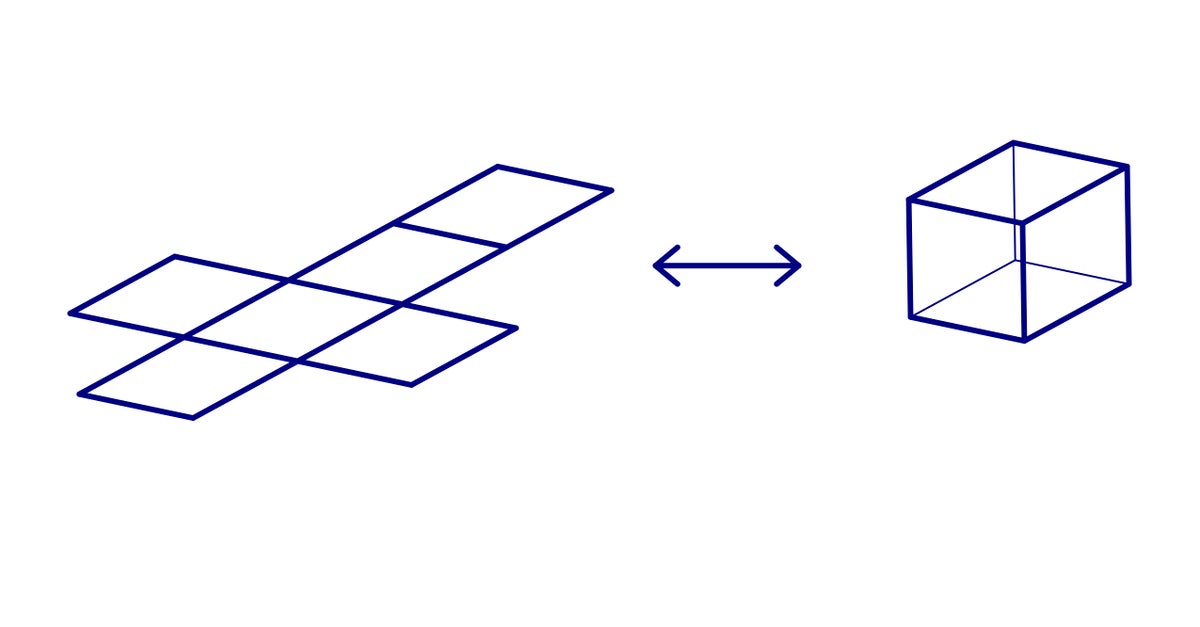
Figure 17. The net of six squares on the left folds up to form a cube; conversely, by cutting appropriate edges you can unfold the cube into the net. (Drawn with Geometer's Sketchpad.)
Look again at the polyhedron in "Melencolia" (Fig. 2). Can you draw a planar net for it, similar to the one shown here for the square? Is your net unique, or can you make it another way? How many different nets can you make for it—how many ways can this polyhedron be cut and "unfolded"? Can every polyhedron be unfolded to a net that lies flat and doesn't overlap itself? This question, which has applications from biology to industrial design, is an important unsolved geometry problem of our time.
Move up one dimension again, this time from three to four. The eight large cubes forming the cross in Figure 18 are a three-dimensional net of a four-dimensional cube! (Yes, the artist, Salvador Dal, knew this; hence "hypercubus" in the title.) What would this net look like, in four dimensions, if you could fold it up?

Figure 18. Crucifixion (Corpus Hypercubus), Salvador Dalí, 1954. Oil on canvas, 76½ × 48¾ inches. Gift of The Chester Dale Collection, 1955. Accession number 55.5. Currently on display in Gallery 913. ©2015 Salvador Dalí, Fundació Gala-Salvador Dalí/Artists Rights Society (ARS), New York.
Space and Time
You'll findtimekeeping devices tucked away in works of art throughout the Met (for example, the hourglass on the wall in Fig. 2.) The Met also contains rich collections of astronomical instruments and timekeeping devices.
In wing of the Art of the Arab Lands, we find beautifully wrought astrolabes. These ancient devices for locating and predicting the positions of the sun, moon, planets, and stars were "the slides rules of the Middle Ages." (You can learn why on the Internet; search, for example, for astrolabes onHowStuffWorks.com.)

Figure 19. Left: Astrolabe of Prince ‘Umar ibn Yusuf. Yemen, 1291 ce. Edward C. Moore Collection. Bequest of Edward C. Moore, 1891. Accession number 91.1.535a–h. Right: Planispheric astrolabe of Muhammad Zaman, Iran, Mashhad, 1654–1655 ce. Accession number 63.166a–j. http://www.metmuseum.org/.
The astrolabe of ‘Umar ibn Yusuf ibn ‘Umar ibn ‘Ali ibn Rasul al-Muzaffari, shown in Figure 19 (left), dates to 1291 ce. It was manufactured in Yemen of cast bronze and then was hammered, pierced, chased, and eventually inlaid with silver to produce a handsome instrument measuring a little more than six inches in diameter. Befitting his royal status and before ascending to the throne, Prince ‘Umar ibn Yusuf had been carefully educated, was well versed in mathematics and astronomy, and had even written a treatise on the construction of astrolabes. Another Met astrolabe (Fig. 19, right), manufactured nearly 400 years later in Mashhad, Iran, by Muhammad Zaman al-Munajjim al-Asturlabi, is made of cast and hammered brass and steel and measures less than 7 inches in diameter. Neither of these astrolabes was large enough to make precise astronomical observations, but they were accurate enough to tell time and could be used to determine the positions of planets for astrological purposes.
Returning to the European Decorative Arts, we find an unusual portable sundial that also served to calibrate sundials (Fig. 20). Made of brass and silver, this object was constructed sometime between 1690 and 1708. The inscription in French, “Cost appliqu au mur pur avoir la declinaison des plans,” indicates its usefulness for determining the declination of the planets. In addition to marking time or determining longitude, this device included a recessed compass for precise orientation.

Figure 20. Combination Portable Sundial and Instrument for Calibrating Sundials. Brass and silver. Left: As seen from the top down. Right: View looking horizontally with the magnetic compass in the rear left; on the right is the gnomon, which would have cast a shadow telling the time at a given latitude. Gift of Mrs. Stephen D. Tucker, 1903. Accession number 03.21.17. http://www.metmuseum.org/.
As clocks became ever more accurate and ubiquitous, sundials actually increased in importance as a means of setting and regulating them (Chandler and Vincent 1967). “Dialing”—or the art of constructing sundials—was a skill that often went hand-in-hand with the work of compass makers and clockmakers. Next to the sundial shown in Figure 20 is a miniature sundial with Roman numerals (Accession number 03.21.60).
The Gubbio Studiolo
That's surely enough for one tour, you may be thinking. But don't leave the Met without visiting the Gubbio Studiolo (little studio) of the Grand Duke Federico III da Montefeltro (1422–1482), sold to and reinstalled in the Met in 1939.
The Grand Duke was not only a successful mercenary general and Papal Gonfaloniere, he was a humanist admirer of the arts, literature, science, and mathematics. Entering his studiolo, you step centuries back in time (Fig. 21). Pale light suffuses the room from a window as on a crisp autumn morning, and the walls of the little study are inlaid with precisely cut pieces of wood fitted together to give the illusion of cabinets and bookshelves full of items wondrous to the eye and mind.

Figure 21. The Gubbio Studiolo (little studio) of the Grand Duke Federico III da Montefeltro. Designed by the Sienese master Francesco di Giorgio Martini (1439–1501); executed ca. 1478–1482 in the Florentine workshop of Giuliano da Maiano (1432–1490) and Benedetto da Maiano (1442–1497). Walnut, beech, rosewood, oak, and other fruitwoods; purchased through the Rogers Fund, 1939. Accession Number 39.153. http://www.metmuseum.org/.
The Studiolo is a meticulously designed example of the spectacular three-dimensional effects that mathematical perspective has made possible. One of the earliest technical treatises devoted to the subject, De prospectiva pingendi (On Perspective for Painting, ca. 1475), was written by one of the earliest masters of perspective techniques, Piero della Francesca (ca. 1415–1492). Piero was among the beneficiaries of Montefeltro patronage, and he dedicated De prospectiva pingendi to the Duke. Another mathematician who received patronage from the Montefeltros was Fra Luca Pacioli (1447–1517), a page of whose famous Summa de arithmetica, geometria. Proportioni et proportionalita we saw in Figure 3. This book, too, is dedicated to Guidobaldo da Montefeltro.
On your visit to the Studiolo (with children or not), see how many items you can find in the room’s many panels that are related to mathematics. Some are obvious, such as the armillary sphere and quadrant, an architect’s plumb bob and carpenter’s square, a compass, and a pair of dividers. Others are perhaps less so, such as the hourglass for measuring time, and the musical instruments that reflect the Duke’s interest in the mathematics of music as one of the liberal arts. The cittern with its nine strings, bridge, and pegbox ‘‘brings to mind the theory of music’s harmonic proportions. During the 15th century the concept of musical harmony and the principles of linear perspective and architectural proportions came to be seen as expressions of the same mathematical truth’’ (Raggio 1999). The largest musical instrument depicted in the Gubbio paneling is a portative organ, just to the right of the door, and the different lengths of the organ’s pipes are clear reminders of the link between the measured proportions and the musical harmonies that the instrument produces when played.
Note too the checkerboard-patterned torus "on" the "table" in Figure 22! Although of mathematical interest, this is not a mathematical instrument. It's a Renaissance mazzocchio, a hat especially popular in Florence in the late 15th century.

Details of Figure 21.
Piero found the mazzocchio particularly well suited for didactic exercises in perspective drawing. The proper rendering of the mazzocchio using mathematical perspective is a notable exercise in his De perspective pingendi, where in Book 3 he uses it to explain the mathematical method of proportionally foreshortening column bases and capitals whereby they may be realized in terms of the principles of perspective (Raggio 1999). In the Gubbio Studiolo, the representation of the mazzocchio was both a test of the mathematical skill of the designer and a mark of the craftsmen to fool the eye and to trick the mind.
Before leaving the Studiolo, take one last look and imagine the Duke da Montefeltro here, in a moment of silence, perhaps pondering one of the mathematical manuscripts Piero had written and had dedicated to him. Two of the musical instruments provoke an odd question: why are some of the strings of the lute and harp broken? Not for lack of attention or maintenance; note that below the harp is a finely wrought tuning mechanism, to keep it in perfect mathematical tune. The Duke would have been aware of the physical and philosophical link between the mathematics and music that is represented throughout the Studiolo, the link that Plato emphasized in the Phaedo, recounting the final hours of the life of Socrates, wherein the strings of the lyre represent harmony, invisible, incorporeal, divine. Yet the lyre is a physical object, and thus the strings are also material, visible, of this world. The broken lute strings, it has been suggested, may be a physical reference to the death of the Duke and his wife, the Studiolo itself not having been completed until shortly after the Duke’s death. In any case, one cannot leave the Gubbio Studiolo without a sense of the peace and harmony of the mathematics that the Duke himself must surely have felt here.
WORKS AND WEBSITES CITED
Carter and Carnarvon 1912. Howard Carter and the Earl of Carnarvon. Five Years’ Explorations at Thebes, A Record of Work Done 1907–1911. London: Oxford University Press, 1912.
Finkel 2007. Irving Finkel. Ancient Board Games in Perspective. London: British Museum Press, 2007.
Jamnitzer 1568. Wenzel Jamnitzer, Perspectiva Corporum Regularium. Facsimile reproduction by Akademische Druck u. Verlagsanstalt, Graz, 1973.
Krumrinie 1997. Mary Louise Krumrine. “Les ‘Joueurs de cartes’ de Czanne: Un jeu de la vie,” Czanne aujourd'hui. Paris, 1997.
Karl Menninger, Number Words and Number Symbols: A Cultural History of Numbers, New York, NY: Dover Publications, 1992.
Raggio 1999. Olga Raggio. The Gubbio Studiolo and Its Conservation. I. Federico da Montefeltro's Palace at Gubbio and Its Studiolo. New York, NY: Metropolitan Museum of Art, 1999.
Harvard Semitic Museum 2006. “Throwing Bones: The Game of 20 Squares,” Video presentation created for the exhibition Houses of Ancient Israel; Domestic, Royal, Divine, Cambridge, MA: Harvard Semitic Museum, 2006: http://semiticmuseum.fas.harvard.edu/games.
http://electronics.howstuffworks.com/gadgets/clocks-watches/astrolabe.htm.
http://engineering.nyu.edu/events/2014/05/17/500-years-melancholia-mathematics).
http://archaeologicalmuseum.jhu.edu/the-collection/object-stories/archaeology-of-daily-life/childhood/knucklebones/.
FURTHER READING
Numerals
Keith Devlin, The Man of Numbers: Fibonacci's Arithmetic Revolution. New York, NY: Walker & Co, 2011.
Seymour Block and Santiago Tavares, Before Sudoku: the World of Magic Squares, New York, NY: Oxford University Press, 2009.
David Eugene Smith. History of Mathematics. Boston, MA: Ginn and Co., 1923–1925, vol. 2: “Finger Reckoning”: 196–202.
Burma P. Williams and Richard S. Williams, “Finger Numbers in the Greco-Roman World and the Early Middle Ages,” Isis 86 (4) (1995): 587–608.
Games
Athanassoglou-Kallmyer 2003. Nina Maria Athanassoglou-Kallmyer. Czanne and Provence: The Painter in His Culture. Chicago, IL: University of Chicago Press, 2003.
Chen and Ankenman 2006. Bill Chen and Jerod Ankenman. The Mathematics of Poker. Pittsburgh, PA: ConJelCo, 2006.
Diaconis and Keller 1989. Persi Diaconis and Joseph B. Keller, “Fair Dice,” The American Mathematical Monthly, 96 (4) (1989): 337–339.
Dunn-Vaturi 2014. Anne-Elizabeth Dunn-Vaturi. “Twenty Squares: An Ancient Board Game,” Exhibition Blog: Assyria to Iberia at the Dawn of the Classical Age. Metropolitan Museum of Art, posted Tuesday, December 9, 2014.
http://www.metmuseum.org/exhibitions/listings/2014/assyria-to-iberia/blog?auth=Anne-Elizabeth+Dunn-Vaturi&st=author.
Jones 1999. Alexander Jones. Astronomical Papyri from Oxyrhynchus. Vols. I and II. Philadelphia, PA: American Philosophical Society, 1999.
Packel 2006. Edward W. Packel. The Mathematics of Games and Gambling. Washington, DC: Mathematical Association of America, 1981; 2nd ed. 2006.
Thorp 1985. Edward Thorp. The Mathematics of Gambling. Secaucus, NJ: Lyle Stuart, 1985.
Dimensions
Abbott 1884. Edwin Abbott, Flatland: A Romance of Many Dimensions. London: Seeley and Co., 1884. See in particular the annotated version by Ian Stewart: The Annotated Flatland. Toronto, Canada: Perseus Publishing, 2002.
Joseph O'Rourke,How to Fold It: The Mathematics of Linkages, Origami, and Polyhedra, Cambridge: Cambridge University Press, 2011.
Space and Time
Chandler and Vincent 1967. Bruce Chandler and Clare Vincent. “A Sure Reckoning: Sundials of the 17th and 18th Centuries.” The Metropolitan Museum of Art Bulletin 26 (4) (1967): 154–169.
Lennox-Boyd, Mark. Sundials: History, Art, People, Science. London: Frances Lincoln Ltd., 2006: esp. pp. 48–85.
Vincent, Clare. “Magnificent Timekeepers: An Exhibition of Northern European Clocks in New York Collections.” The Metropolitan Museum of Art Bulletin 30 (4) (1972): 154–165.
The Studiolo
Cheles 1986. Luciano Cheles. The Studiolo of Urbino: An Iconographic Investigation. University Park, PA: Pennsylvania State University Press, 1986.
Raggio 1996. Olga Raggio. “The Liberal Arts Studiolo from the Ducal Palace at Gubbio,” The Metropolitan Museum of Art Bulletin 53 (4) (Spring, 1996): 5–35.
Rotondi 1969. Pasquale Rotondi. The Ducal Palace of Urbino: Its Architecture and Decoration. London: Alex Tiranti, 1969.
Wilmering 1999. Antoine M. Wilmering. The Gubbio Studiolo and Its Conservation. II. Italian Renaissance Intarsia and the Conservation of the Gubbio Studiolo. New York, NY: Metropolitan Museum of Art, 1999.
If You Go
The Met's address is 1000 Fifth Avenue (at 82nd Street), New York, NY 10028, USA (see Fig. 24). The museum is open 7 days a week. Check the Met's website for opening hours and other information.
Map your route carefully; the museum is vast. You can download a map of the Met from the website or can pick one up at the information desk in the Museum’s entrance hall. Note: The last stop on this tour, the Gubbio Studiolo of the Grand Duke Federico III da Montefeltro, is permanently installed in Gallery 501, but nevertheless it is not easy to find. Entering the Museum from Fifth Avenue, continue straight ahead either to the right or to the left of the grand staircase leading to the European portrait galleries on the second floor. Instead of taking the staircase, follow the first-floor corridors of medieval objects on either side until reaching the Spanish courtyard, whereupon, turning right, the main corridor leading to the hall of medieval armor takes you into a small vestibule, and immediately on entering this, turn right to find the doorway leading into the Studiolo.
This article from The Mathematical Intelligencer was reprinted with permission. It was originally published in September 2015.
